The Arbitrage Pricing Theory or APT is not only one of the most basic theories of finance, it is one of the theories that is closest to being true. The theory itself gives a reason the APT is so close to being true: because it depends primarily on the principles of arbitrage and diversification being applied by some investors. So the APT is robust to large numbers of investors with a huge amount of investable wealth behind them being irrational–the “noise traders” of the post’s title–as long as there is a remnant of rational investors who also command a significant amount of investable wealth. 

In this post, I want to explain what I see in the APT at the level I do finance in my undergraduate “Monetary and Financial Theory” class. In particular, I am assuming the level of knowledge in my handout “Notes on the Capital Asset Pricing Model.” You can see another take on the APT in the Wikipedia article on Arbitrage Pricing Theory, and I would be glad for recommendations for other links to especially accessible and well-written treatments of the APT. 

To simplify, I am thinking about real returns over a short period of time, coming out of the usual jagged path of prices without sudden jumps. And I am leaving aside issues of intertemporal hedging so that everyone’s objective boils down to a function of only the mean and variance of the overall portfolio returns over that short span of time. (Key issues this leaves out are intertemporal hedging, which Matthew Shapiro, Tyler Shumway and Jing Zhang talk about as the issue of appropriate numeraire for each age by risk tolerance group by which to evaluate returns in our paper “Portfolio Rebalancing in General Equilibrium” and integration of human capital into portfolios.)   

The returns for different assets often covary, either positively or negatively. Some of this return variation and covariation might be due to the actions of the noise traders. Indeed, the returns due to variation in the required return for risky assets are a good candidate for noise-trader-induced return variation and covariation. 

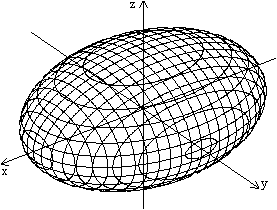
If the way the return of each asset varies is thought of as a vector, then two assets that covary positively–that is, move together to some extent–can be thought of as vectors with an acute angle (of less then 90 degrees) between them. Two assets that covary negatively can be thought of as two vectors with an obtuse angle (of greater than 90 degrees) between them. Two assets that are uncorrelated can be thought of as two vectors that are orthogonal to one another–that is, two vectors that have a right angle of 90 degrees between them. From this point of view, all the variation and covariation of all of the assets out there can be thought of as an ellipsoid with many, many dimensions. 

To give you a little of the picture of what an ellipsoid in many dimensions is like, let me quote in full the text of my post “My Proudest Moment as a Student in Ph.D. Classes”: 

Ellipsoids, which are more or less a watermelon shape, are important in econometrics. In my Ph.D. Econometrics class at Harvard, Dale Jorgenson explained the effect of linear constraints by saying that slicing a plane through an ellipsoid would be like slicing a watermelon. Slices of a 3-dimensional ellipse–a watermelon–are in the shape of a 2-dimensional ellipse–a watermelon slice. Dale’s analogy of watermelons and watermelon slices inspired me to exclaim that slicing a 4-dimensional ellipsoid with a hyperplane would get you a whole watermelon! 

No matter how many dimensions are in play, except in rare cases of equal lengths, there will be one major axis (usually at a slant) along which the ellipsoid representing all the asset returns is the longest. In the APT, this represents the “first factor” or most important way in which the universe of assets covaries. Then, among all the directions at right angles to that, the longest axis is the “second factor” and so on. When the covariances of the returns are analyzed, it turns out that after a few factors (each at right angles to all the previous factors), the ellipsoid is pretty thin in all the directions at right angles to the first few factors.

Suppose that each of these factors is made into a hedge fund or mutual fund. If we go through all the factors, even the minor ones, and make them each into a hedge fund or mutual fund, these hedge funds and mutual funds (hereafter “hedge funds”) will cover all the bases and make any position possible that was possible with the full set of assets.

Imagine that there is a set of rational investors who all agree on these covariances and on the mean excess returns that each asset earns above the safe rate. These investors may differ in their risk aversion. I am going to leave aside any rational investors who are ever limited from short-selling or from borrowing to take a more than 100% position in risky assets overall, and focus on a core group of rational investors who are never limited in those ways.

Asset Demand by the Unconstrained Rational Investors 

Each unconstrained rational investor k will want to choose a share in each of the factor funds obeying the following equation:

share of wealth in factor fund i 

= risk tolerance of k * mean excess return of i / variance of i 

Here, risk tolerance is just a name for 1/relative risk aversion. Risk tolerance turns out to be a very useful concept because risk tolerance averages across people as a simple wealth-weighted mean, while risk aversion correspondingly averages across people as a more complex harmonic average. To see this note that 

total holdings of factor fund i by all unconstrained rational investors =

sum over unconstrained rational k of wealth of k * risk tolerance of k 

* mean excess return of i / variance of i.

It is convenient to define aggregate risk tolerance of unconstrained rational investors by 

aggregate risk tolerance of unconstrained rational investors

= sum over unconstrained rational k of 

(wealth of k / total wealth of core rational investors) * risk tolerance of k.

Then dividing the equation just before that by the total wealth of all unconstrained rational investors, 

share of the wealth of all unconstrained rational investor in factor fund i 

= aggregate risk tolerance of unconstrained rational investors  

* (mean excess return of i / variance of i). 

Setting Supply Equal to Demand

Think of the supply of each asset left over for the unconstrained rational investors as if it were exogenous. At any rate, however endogenous that is, since someone must hold all the assets that exist, whatever assets are left over for the unconstrained rational investors must be held by them as a group. I will represent the key aspects of the supply of assets left over for the unconstrained rational investors by the shares of the various factor funds in the total pile of wealth that the unconstrained rational investors as a group hold. 

It is natural to define the aggregate risk aversion of the unconstrained rational investors as 1 / aggregate risk tolerance of unconstrained rational investors. Given that definition, after setting the shares in the supply left over for the unconstrained rational investors to the share on the demand side for the unconstrained rational investors, a little algebra gives a formula for the mean excess return of factor fund i when the 

mean excess return of i 

= aggregate risk tolerance of unconstrained rational investors 

* variance of i 

* share of i in what is left over for unconstrained rational investors.

The interesting thing about this equation is that it has the key asset pricing prediction for the APT: that the mean excess return is almost zero for a minor factor (e.g. the 23d factor) for which the factor fund has almost no variance after the diversification implicit in forming the i-th factor fund. 

Among factors that have some variance even after diversification, the mean excess return will be higher when the unconstrained rational investors are asked to hold a lot of it–as well as, of course, factors that have a high variance. 

The Covariance with the Unconstrained Rational Investors’ Portfolio

Consider an unconstrained rational investor k. Let the risk aversion of investor k be Ak. Call the excess return of this investor’s whole chosen portfolio (including whatever safe assets it contains) yk. Let h be the amount of any asset with excess return x more or less than what investor k actually chooses. By the definition of h as a perturbation, the optimal level of h is 0. That is:

h* = 0.

Because of the simplifying assumptions (including continuous time), the investor maximizes a mean-variance expression:

{max over h} E ( yk + hx) - (Ak/2) Var( yk+ hx).

Using a little statistical algebra, including expanding the variance as one would the square of a sum, this optimization problem becomes

{max over h} E (yk) + h E(x) - (Ak/2) Var( yk) - h Ak Cov(yk,x) - (h2/2) Ak Var(x).

(h2 here is just h squared.) As a function of h, this is a smooth parabolic hill. The easiest way to find the top of the hill–the optimal value of h or h*–is to use the fact that the top of a smooth hill has a zero slope. Taking the derivative with respect to h and writing that it is zero at h*, 

E(x) - Ak Cov(yk,x) - h* Ak Var(x) = 0.

But because h* was a disturbance or perturbation away from what the rational investor chose to do, h*=0. Thus, after a tiny bit of rearrangement: 

E(x) = Ak Cov(yk,x)

or

( E(x) / Ak ) = Cov(yk,x).

If zk is the fraction of all the wealth of unconstrained rational investors held by investor k, then 

sum over unconstrained rational k of [zk ( E(x) / Ak )]

= sum over unconstrained rational k of [zk Cov(yk,x)].

This boils down to the very interesting equation

aggregate risk tolerance of unconstrained rational investors * E(x)

= Cov(y,x),

where y is the excess return of the aggregate portfolio (including any safe assets) of all unconstrained rational investors put together. Equivalently, for any asset with excess return x,

E(x) = aggregate risk aversion of unconstrained rational investors * Cov(y,x).

That is, with supply equated to demand, the excess return of any asset must be proportional to the covariance of that asset with excess return of the pile of assets that have been left over for the unconstrained rational investors. This is true even if most investors are noise traders, as long as there is a core group of unconstrained rational investors. The constant of proportionality is the aggregate risk aversion of those rational investors. 

The Capital Asset Pricing Model

The Capital Asset Pricing Model–or CAPM–is the special case of the APT in which all investors are unconstrained rational investors. Then the assets left over for the unconstrained rational investors is simply the universe of all assets (including any safe assets), and the mean excess return of any asset is proportional to the covariance of that asset with the portfolio composed of the universe of all assets. And the constant of proportionality is equal to the aggregate risk aversion of all investors. 

One nice thing about this proposition is that if there are some households that avoid risky assets, but all investors who do invest in risky assets are rational, then all risky investors are rational, and the logic above implies that the CAPM will still hold (since the covariances only depend on the risky assets), except that the constant of proportionality is their aggregate risk tolerance only if the safe assets held by the households that (perhaps irrationally) do not invest in risky assets are excluded.

Note that if investors cannot see through the government veil so that Ricardian equivalence and Wallace equivalence totally fail, then the unconstrained rational investors would be simply the private investors and the covariance that matters is with the portfolio of private investors and the aggregate risk tolerance is that of the private investors. 

Note on the Integration of Human Capital and Houses into the Rational Investors’ Portfolio

If the unconstrained rational investors integrate human capital into their portfolio decisions, that complicates things. One simple way to modify the propositions above to finesse that issue is to focus on unconstrained, rational, retired investors and to treat their social security wealth as a safe asset.

Their houses should be included in the aggregate portfolio of these investors, however, with the caveat that because of the frictions making it difficult to get a little more or a little less house, the covariance condition may not apply to the houses themselves, though it should apply to other more easily variable assets with the covariance including, in part, the covariance with those houses.

Intertemporal hedging is then one of the biggest remaining issues.

Note that even if everyone is rational, focusing on the covariance of an asset with the aggregate portfolio of retired investors could be helpful in order to avoid concerns about human capital.